Transition from Wigner crystal to Wigner molecule
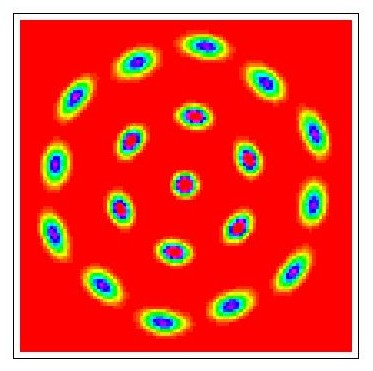
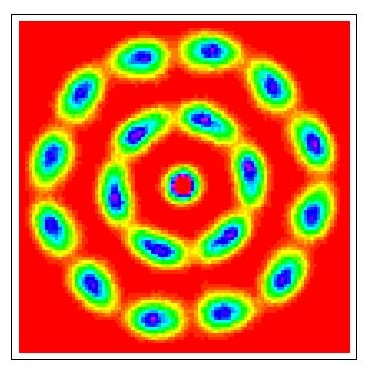
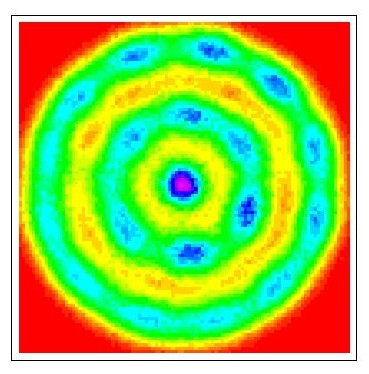
Picture shows three snapshots of the arrangement of N=19 electrons at low temperature.
The electron probabilty is high at purple spots and decays via green and yellow to zero (red).
From left to right, the density (confinement strength) is increased, giving rise to increasing overlap of the neighboring electron clouds.
Notice the regular symmetry of this cluster: with 1, 6 and 12 electrons on the three shells each electron is part of (at least one) triangular lattice cells.
This cluster is, therefore, called "magic", it has unusually high stability against inter-shell rotation, see the figure below.
right figure:Wigner molecule - angular and radial wave function overlap (further density increase leads to a Fermi liquid state)
center figure:Wigner crystal - only angular wave function overlap. Electrons cannot move between shells, but shells can rotate as a whole.
left figure:completely (radially and orientationally) ordered Wigner crystal - practically no wave function overlap. No shell rotation is possible.
Notice that the shells are not round as in the right figure, but have an almost perfect hexagonal shape. Each particle is involved in an triangular lattice cell.
The same pictures as nice little animation
Wigner crystal in the temperature-density plane
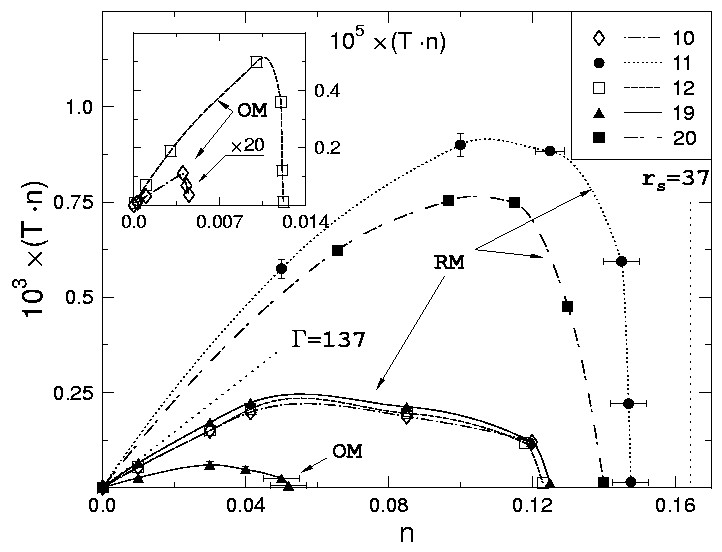
Phase boundary of the Wigner crystal for various particle numbers. "RM" denotes radial melting, i.e. transition to a Wigner molecule. "OM" stands for orientational melting, which denotes the transition between the radially and orientationally ordered phase and the orientationally disordered crystal (where the shells can rotate with respect to each other).
Notice the huge difference between the melting curves for various particle numbers (for exmaple for N=19 compared to N=20)
see the article of A.V. Filinov, M. Bonitz, and Yu.E. Lozovik, just published in Phys. Rev. Lett. vol. 86, p. 3851 (2001)
This work is featured by the American Physical Society's Physical Review Focus, article of April 19 2001 by Geoff Brumfiel
This work is featured by "Wissenschaft Online", article of April 24 2001 by Thorsten Krome (Deutsch)